8 Popular Common Core Math Standards Explained with Examples in the Classroom

The dust has finally settled, and it looks like Common Core math is here to stay.

After countless political battles (and more than one Common Core math meme floating around social media), the initiative that incorporates techniques like cooperative learning and active learning has settled into the American education system.

Prodigy offers common Core-aligned math practice that your students will love. Start today!

Beginning in 2010, the Common Core State Standards Initiative(CCSSI) aimed to change the way American students were taught English language arts and mathematics by countering low test scores, inconsistent learning standards and a curriculum that was a “mile wide and an inch deep.”

Of the 45 states (plus the District of Columbia and the Department of Defense Education Activity) that fully implemented Common Core by 2015, 24 chose to revise some aspects of the program but still remain aligned with the original standards today.

What is Common Core math?

The Common Core State Standards for Mathematical Practice were designed to reform the American education system, with three main goals:

1. Provide graduating high school students with the skills they need to be successful either in the workforce or in post-secondary education
2. Boost math test scores for all American students
3. Smooth out the differences between individual state curriculums and practices

At the heart of Common Core math are the eight Standards for Mathematical Practice. These standards were created by education professionals at all levels, and are based on research, leading state curricula and exceptional international math programs.

1. Make sense of problems and persevere in solving them
2. Reason abstractly and quantitatively
3. Construct viable arguments and critique the reasoning of others
4. Model with mathematics
5. Use appropriate tools strategically
6. Attend to precision
7. Look for and make use of structure
8. Look for and express regularity in repeated reasoning

These standards allow students to learn deeply instead of widely and build a solid foundation for advanced study. The traditional Common Core math provides guidelines for grade-specific concepts, but it’s up to individual school districts to implement a curriculum that’s in line with the standards.

Keep reading to find out what they mean, or download our free, condensed list of the eight standards and examples for teaching them!

Is Common Core math working?

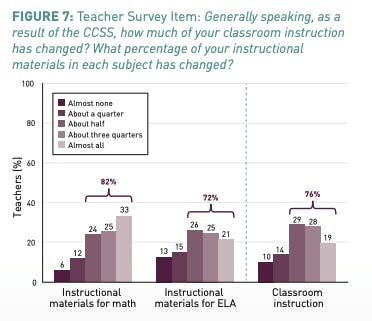
Due to the massive overhaul of state education frameworks, many teachers are still scrambling to prepare. A study from the Center for Education Policy Research at Harvard University reported that82%of math teachers are changing “more than half of their instructional materials” in response to the new practice standards. The same study found that “three out of four teachers (73%) reported that they have embraced the new standards ‘quite a bit’ or ‘fully’.” 

Source: Center for Education Policy Research

1. Make sense of problems and persevere in solving them

When students approach a new problem for the first time, they might be tempted to go straight for the solution. After all, isn’t that the point? The first standard directly counters this impulse.

When students rush in to immediately solve a problem, they often fail to understand the underlying concepts. Rote memorization and a quick recall are essential parts of mathematical fluency, but can often lead to greater problems. If a student doesn’t understand the underlying concept behind the facts they’ve spent time learning, they might struggle with more complicated problems or ideas.

Giving students more open-ended questions or methods allows them to work with the concepts behind the problem, instead of going straight to the solution.

For example, look at this typical Common Core math problem:

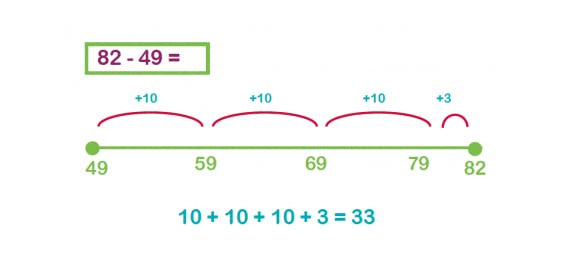
Source: The School RunWhile it looks complicated, a number line is a Common Core math example that teaches students several essential concepts:

• The relationship between numbers in a given problem
• The potential for more than one solution
• The foundation behind shortcuts and more complex processes

Example:

Jennifer Smith and Michelle Stephan used this question to incorporate the first standard into a seventh-grade classroom:

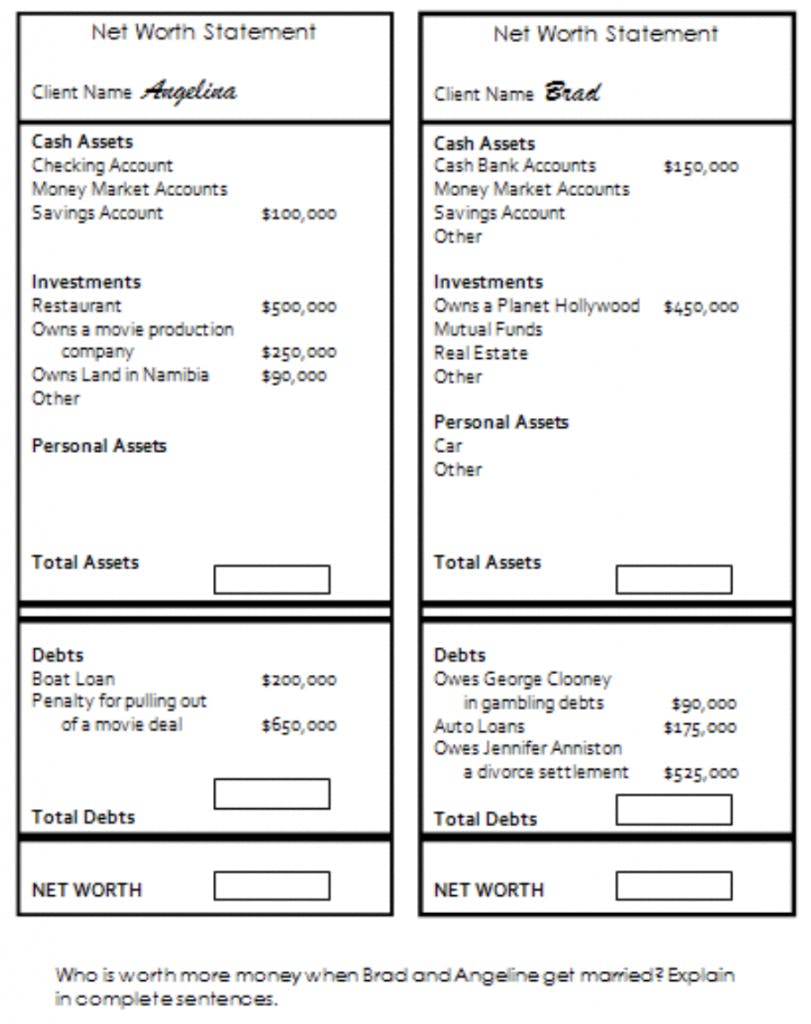
Source: Journal of the American Academy of Special Education Professionals

The problem was presented to the class with a quick introduction, and the teachers asked students to find the greater net worth -- without explaining how. Students worked alone or in groups to discuss the question and their process, while the teachers supervised and made note of different strategies.

The teachers spent minimal time with the students, fixing only minor mistakes and encouraging them to work with their group. Every student was actively engaged in working with the problems, and explained their thinking to the class in a follow-up discussion.

2. Reason abstractly and quantitatively

There are two parts to the second standard: decontextualization and contextualization.

Decontextualization refers to the process of understanding the symbols in a problem as separate from the whole. This is where the much-loved word problem becomes essential.

Take this question as an example:

Decontextualization means a student is expected to infer from the above problem that they are to solve an equation (5 + 3 = 8) without getting distracted by any additional information.

Contextualization is the opposite: it refers to the ability to step back from the problem and view it as a whole. Students would have to understand that five bouquets of flowers represent the total amount, and the three more that Steve brings are adding to that original number.

Example:

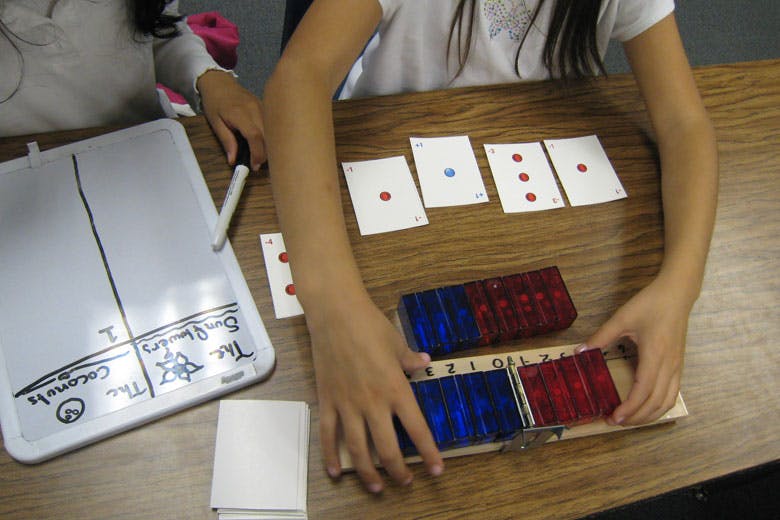
Source: Stanford Graduate School of Education

A study conducted by the Stanford Graduate School of Education found that the same parts of the brain that compare physical size also compare the abstract worth of two numbers. By linking those two processes using modular tools, the study found that students were better equipped to learn about abstract concepts like negative numbers, negative fractions and pre-algebraic problems.

Researchers used different colored blocks to represent negative and positive numbers, and asked students to find the midpoint between two sums. In your classroom, have younger students model addition and subtraction with number blocks, or ask older students to find the dimensions and volume of an everyday object using the formulas they’ve learned.

3. Construct viable arguments and critique the reasoning of others

Are your students just repeating the steps without understanding what they’re actually doing, or are they building the strong theoretical foundations they need to tackle high-school- and college-level problems? Similar to the first standard, this standard encourages critical thinking and problem-solving.

Challenging your students to look at data, solve problems, draw conclusions and debate with their classmates is a great way for them to ask new questions and develop a solid understanding of definitions and processes.

Example:

The best way to develop the third standard is through structured classroom discussion. Before you start working on the solution to a problem with your class, brainstorm some strategies: Put the simplest answers on the board first, then move on to more advanced strategies. Talk through each strategy as a group, and discuss what was right or wrong about the approach.

Some more tips for having a great classroom discussion:

• Ask students to write down answers to questions, like “what did you find difficult about this problem?” or “what did you learn during this activity?” before sharing out loud
• For a more lively class, consider implementing a “talking stick” or other object to let students know who has the floor
• Read our post for more ideas on effective classroom management

4. Model with mathematics

Different types of learners respond best to different instruction styles, and it can be difficult to respond to the personalized learning needs of each student.

However, many different types of learners respond well to seeing their textbooks brought to life. That’s exactly what high school math teacher Dan Meyer illustrates in this TED Talk:

It’s not just teachers who can show students the real-world applications of math. Reversing the processes can have a valuable effect on how students engage with problems and the world around them. Challenge students to take a problem from the page to real life using number lines, diagrams, o rclassroom technology.

Example:

This is also a great time to try out project-based learning strategies, like third grade teacher Renee McFall did with her classroom.

In order to bring math to the real world, she challenged her students to raise money for a local charity by selling bracelets. Students were responsible for making and selling bracelets, calculating the amount of supplies they needed, making a budget, and pitching their best business ideas to teachers. With real-world consequences, students were encouraged to be precise in their calculations, measurements and planning, because mistakes could cost money.

5. Use appropriate tools strategically

Students today have a huge variety of tools available to them, and knowing which to use is half the battle. Depending on the problem, students could use anything from scrap paper and a pencil to more advanced technology resources. When students know how to find what they need, they develop problem-solving skills and become more comfortable looking for new solutions in the future.

Example:

One practical way to get your students familiar with the appropriate tools is to challenge them to figure out what they need themselves. At the beginning of the lesson, ask them to make a list of the tools they need and gather them up.

Some options to include:

• Pencils and paper
• Calculators
• Modular tools
• Worksheets with key formulas

Ask more advanced students to brainstorm a list of sources to draw research from, like books, websites or even podcasts. Afterwards, have a discussion about the tools used. Were there any differences between what students chose? What worked and what didn’t? What tools would they consider using next time?

6. Attend to precision

Precision is one of the most important skills to develop early on in math study. Even if most first graders would rather finger paint than write numbers, it builds a solid foundation for more advanced math problems. Encouraging students to use correct symbols and challenging them to accurately communicate their process to others gets them comfortable with the “language” of math.

In younger grades, students can practice precision by explaining their thinking to classmates, using either words or modular tools. As students get older, they can begin to accurately define units and equations, both in writing and speaking about math.

Example:

Have students start a math journal to practice precision and communication. Younger students can respond to prompts of “what they did” and “what they learned.” Older students can use their journal space to engage more with the topic and ask questions about concepts they don’t quite understand yet.

Write prompts on the board to get your students started:

• Write a letter to a family member explaining your process
• Do you have any more questions you want answered?
• Where did you get stuck in this problem? Why?
• What tools did you use to solve this problem?
• What will you do differently next time?

Be aware that students might need some time to adjust to writing about math. Make sure to model it to the class and provide students with lots of prompts to get them started.

7. Look for and make use of structure

Seeing repeated patterns gives students the tools to reason through new, more challenging problems.

Sean Nank, recipient of the Presidential Awards for Excellence in Mathematics and Science Teaching, defines the understanding of patterns and structure as being key for math fluency:

Structure allows students to understand that complicated equations are not whole entities, but rather composed of several smaller, more accessible objects. This understanding gives them the confidence to attempt more difficult equations.

Example:

One of the best ways to develop an understanding of structure is through a daily math practice. Prodigy is an engaging, game-based math platform aligned with Common Core math curricula. It’s an exciting online resource that challenges students to answer math questions every day as they duel characters, play with their friends and collect exotic pets.

To see an even greater impact on your classroom, use the teacher tools to set assignments that help students build confidence in a particular skill.

Other great options for building a daily math practice include challenging your students to solve a daily math problem when they arrive in the classroom, or setting aside time in your lesson for students to model problems with modular tools so they can see the patterns for themselves.

8. Look for and make use of repeated reasoning

The seventh and eighth standards are closely related, but it’s important to distinguish between them. Instead of focusing on the repeated structure of an object, the eighth standard encourages students to use past problems as a model for present ones.

When students can demonstrate repeated reasoning, it means they’re able to try different solutions for the same problem and adjust as needed. Students can see which elements stay the same and which are variable by testing different methods repeatedly. This process develops both attention to detail and oversight -- controlling the small parts of the problem while making sure that overall, they’re on the right path to a solution.

Example:

A great way to promote repeated reasoning is through the use of “fact families.” When students write an equation, challenge them to write two or three more equations that directly relate to the original, like this:

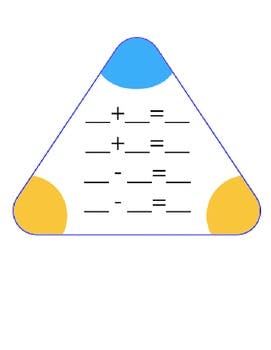
Source: Teachers Pay Teachers

As students progress and become more advanced, this provides a solid foundation for more complicated equations that include fractions, integers and algebraic elements.

Fact families encourage students to maintain a focus on the overall equation, while also manipulating the individual numbers and examining the relationships between them. Working with fact families to express repeated reasoning in early elementary gives students the skills they need for later elementary, high-school level and post-secondary math.

Tips for explaining Common Core math to parents:

All set! You’ve seamlessly integrated Common Core in your classroom, your students are working together and discussing their ideas, and things are going smoothly. But what about their parents?

Parents want their children to get the best education possible. Common Core math is quite a large shift from how they were taught as children, and some of the processes and techniques might be unfamiliar to them.

With that in mind, here are three ways to get parents on board with new Common Core math standards:

1. Send home an information sheet or link parents to a web resource that explains the standards behind Common Core math and how they work in the classroom. Parents are less likely to be alarmed by dramatically different-looking homework when they’ve been given a head’s-up and an introduction to the reasoning behind the change.
2. Let parents know that their children might ask questions and struggle while they get used to the new curriculum. A recent study from Psychological Science found that when parents expressed negative feelings about math, their children were also more likely to underperform. Encourage parents to model a positive attitude and to work through difficult problems with their children.
3. Consider taking a few minutes during a parent evening to go over the most important points of the new curriculum, and encourage them to keep in touch and to reach out if they have any questions. Supporting an open dialogue with parents is a great classroom practice, no matter which subject you’re teaching.

Education doesn’t happen in isolation — in fact, one of the key indicators for students’ success is how engaged their parents are with their schooling. Keep parents in the loop to avoid major frustration and confusion and ensure a positive learning environment for all your students.

Common core math standards: Final thoughts

Such a large shift in curriculum and teaching habits is bound to have a few growing pains, and certainly won’t happen overnight. However, with a little bit of time, patience and hard work, you’ll begin to see confident and engaged learners.

The biggest strength of the Common Core math standards is their versatility -- they overlap and complement each other to ensure all children are confident in their math skills.

“I see the Common Core as a way to provide teachers with strategies,” says Sean Nank, “so that students can see the beauty of math -- the how it works and the why it works and the patterns.” Encourage your students to keep looking for the how’s and the why’s, and watch them flourish.

Create or log in to your free teacher account on Prodigy– an engaging, game-based learning platform for math that’s easy to use for educators and students alike. Prodigy is aligned with curricula across the English-speaking world and filled with powerful teacher tools for differentiation and assessment.